Solitaire Win Rates: All in a Row

All in a Row

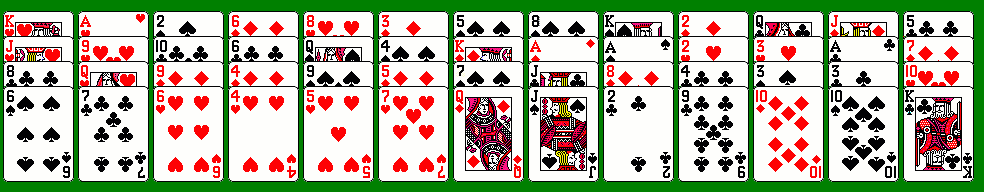
My solver won this game 99.98% of the time. This is far higher than the 67% figure you may have seen quoted elsewhere!

The reason for the discrepancy in win rates is because the other solvers reporting 67% did not play the game using the original rules as described in 1909 by Henry Preble. I assume somebody misinterpretted the rules, and then everyone else (including myself, at first) learned the incorrect rules from each other (or from Wikipedia). Fortunately, ace solitaire sleuth Michael Keller was on the case, and figured out that we were not playing the correct game! See SolSuite for the correct rules.

So, I decided I would be first to write a solver to play the original All in a Row. Actually, it's not quite the same game; it plays the open variation in which you can see all the cards (which I assume is how most people will prefer to play it anyway).

Although I suggested playing the game fully open, in my opinion doing so makes the game too easy to win. One option to make the game more difficult, as mentioned in the original rules, is to disallow "wrapping", so that Kings and Aces cannot connect together in the middle of a discarded set. A different way I thought of (which I prefer) is to require more than two cards to be in each discarded set. I determined the win rate using a range of minimum discard lengths, with and without wrapping allowed.

All in a Row, wrapping allowed
Minimum Discard Length	Deals Played	Games Won	Win Rate %
2	100,000	99,980	99.98
3	10,000	9,501	95.0
4	10,000	6,524	65.2
5	10,000	2,882	28.8
6	10,000	775	7.8
7	10,000	97	1.0
8	100,000	114	0.1
9	10,000,000	233	0.002
10	10,000,000	48	0.0005

All in a Row, wrapping disallowed
Minimum Discard Length	Deals Played	Games Won	Win Rate %
2	100,000	99,692	99.69
3	10,000	6,990	69.9
4	100,000	12,089	12.1
5	1,000,000	2,442	0.2
6	10,000,000	331	0.03

I also have results for the mistaken version of All in a Row (similar to Black Hole) on my Quasar page.

See Michael Keller's Golf and its Variants page for more about Golf variants (including All in a Row).

Any comments are welcome, please send to:

Last modified September 10, 2021

Main page